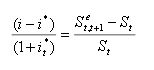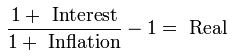The interest rate parity gives a mathematical explanation for the purchasing power parity and real effective exchange rates. It explains why currencies with low inflation must appreciate with time.
Low inflation countries – often called safe-havens – have wages and implicitly inflation, that do not rise as quickly as in other countries. With lower wage costs, profits of companies increase and the current account and the international investment position are positive. This has a repercussion on the balance of payments: More investor inflows into these profitable companies than outflows.
Since longer-term bonds are related to inflation, the government pays less interest on its debt. Thanks to low bond yields, many of the safe-havens are low-tax countries. Examples of those “safe and tax-havens” are Switzerland or Singapore. Germany is a safe-haven since the 1970s, because wages increased more slowly than in other European countries, with the consequence of a strong German Mark. The influence on the balance of payments is slightly different: These inflows into safe government bonds mostly happen during risk-off phases.
The interest rate parity gives the mathematical explanation why such a low inflation currency must appreciate.
Interest Rate Parity
(Most used source for this chapter)
Assuming a forward market exists, the investor can either save at home, receiving interest rate i, or converting by the exchange rate S , receiving interest rate i* abroad, and then converting back to home currency by the forward rate F obtaining at time t for a trade at time t+1.
This condition is called “covered interest rate parity”, reflecting the fact that investors are “covered” against nominal uncertainty by way of the forward market.
If the gross return of foreign investments converted in local currency is higher than the return of investments in the home country, then investors will prefer the foreign currency investments.
If the forward rate is equal to the future spot rate (see also “forward premium”) such that
then we obtain the “uncovered interest rate parity”:
In countries with high inflation, money is not worth always the same. Money in this currency loses real (i.e. inflation-adjusted) value, you can buy less with it after some time Therefore the ex-ante purchasing power parity, suggests that these currencies depreciate over the long-term.
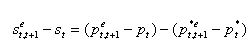 The p terms stand for foreign expected prices minus current prices, i.g. foreign inflation, compared with the p* terms, local inflation.
The p terms stand for foreign expected prices minus current prices, i.g. foreign inflation, compared with the p* terms, local inflation.
On the other side, it is possible that despite higher inflation, a foreign currency does not depreciate. The reason is that investments in this currency yield more. This is reflected in the real interest parity.
Higher yields on investments (“marginal product of capital”) compensate for the fact that inflation is higher.
Since the central banks need to combat high inflation, they are obliged to hike interest rates until they are equal to the yield of investments minus a risk premium. Neoclassical models assume that the marginal product of capital equals the real interest rate, this condition is equivalent to the equalization of marginal products of capital equalized across borders.
Real Interest Rate and Fisher equation
The Fisher equation leads to the definition of real interest rates.
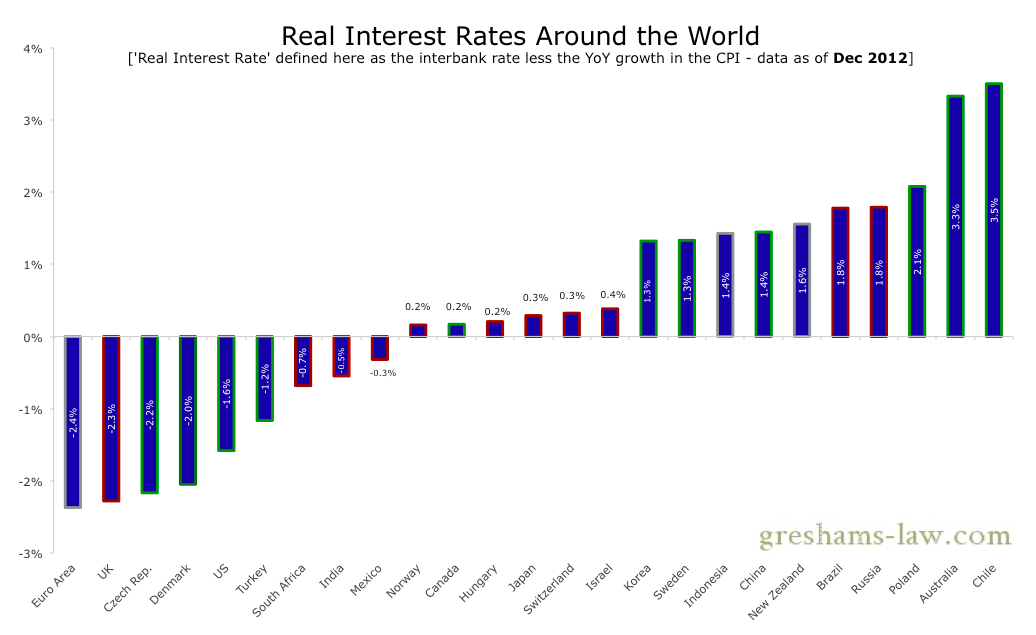
The following graph shows real interest rates around the world based on central bank interest rates as December 2012.
(click to expand)
The mean reversion for to real exchange rates
The uncovered interest rate parity is visible in the mean reversion of currencies to the real (inflation-adjusted) exchange rate.
A pure mean reversion is valid for P/E ratios. The P/E ratio must come back to average Shiller Price Earning Ratio. A strongly performing stock like Apple, must return towards the mean, because the competition is able to produce similar products. Stock prices rise over time according to GDP growth and inflation rate (see the Gordon growth model). Indices are positively influenced by a survivor-ship bias and includes sometimes even dividends (Performance indices).
A mean reversion for currencies does not exist neither: currencies with low inflation and current accounts surpluses must appreciate over the time.
See more for